Homogeneous System Of Linear Equations

Any solution is a linear combination of basis vectors. For example is a system of three equations in the three variables x y zA solution to a linear system is an assignment of values to the variables such that all the equations are simultaneously satisfied. Any higher order linear system of ODEs that can be reduced to one of the 5 forms of systems. Differential equations in the form y pt y gt. X 0 y 0 is always a solution of the homogeneous system of equations with unknowns x and y then which of the following statement is true. Homogeneous system of linear equations is always consistent. A A is true and R is the correct explanation of A b A is false and R is not a correrct explanation of A c A is true and R is.

System of homogeneous linear equations AX 0.

Means all the unknowns has same value as zero. Any higher order linear system of ODEs that can be reduced to one of the 5 forms of systems. We give an in depth overview of the process used to solve this type of differential equation as well as a derivation of the formula needed for the integrating factor used in the solution process. Solving single linear inequalities follow pretty much the same process for solving linear equations. In other words the definition of a control system can be simplified as a system which controls other systems to achieve a desired state. Linear First Order non-constant coefficient homogeneous system of ODEs 4.

Homogeneous Systems Of Linear Equations Youtube

Homogeneous system of linear equations. Homogeneous linear differential equations with constant coefficients. We can also solve these solutions using the matrix inversion method. Those systems of two equations and two unknowns only.

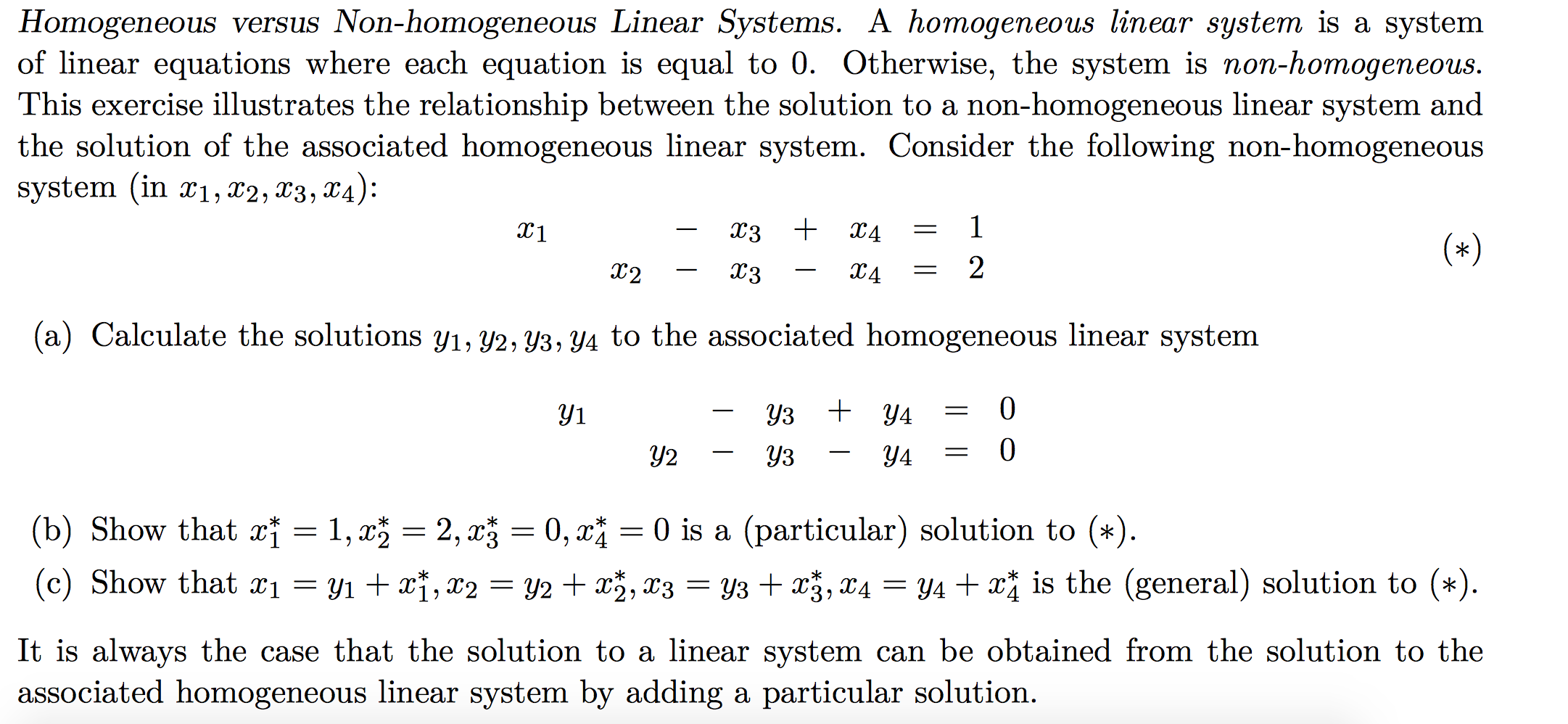
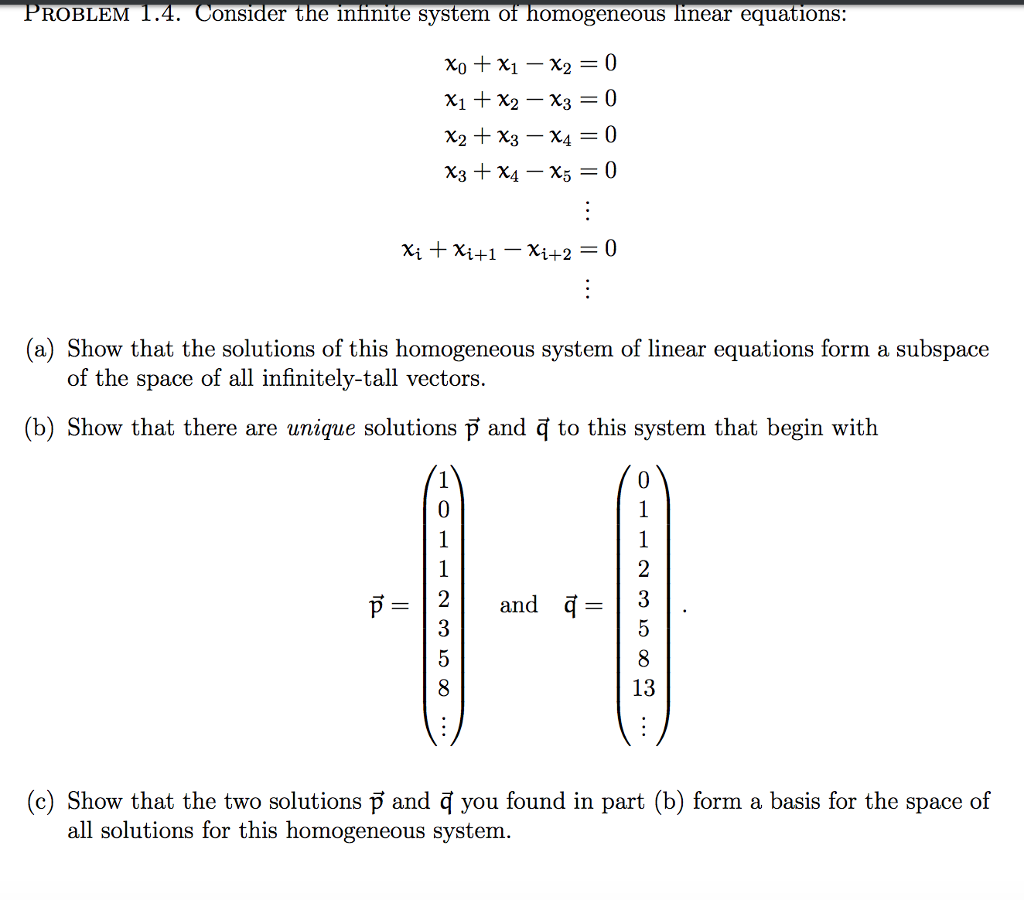
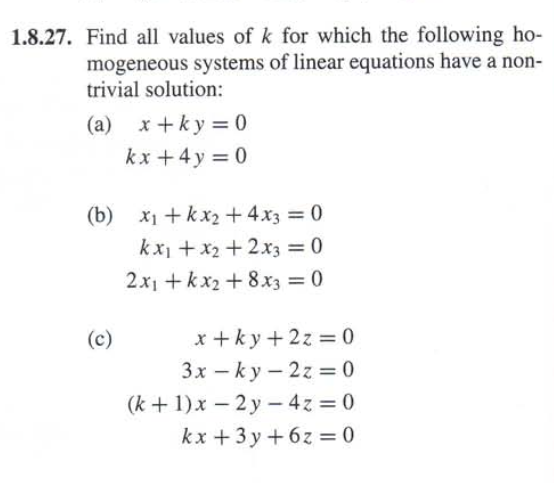
Any other solution is a non-trivial solution. This is also true for a linear equation of order one with non-constant coefficients. A 1n x n g 1 x 2 a 21.

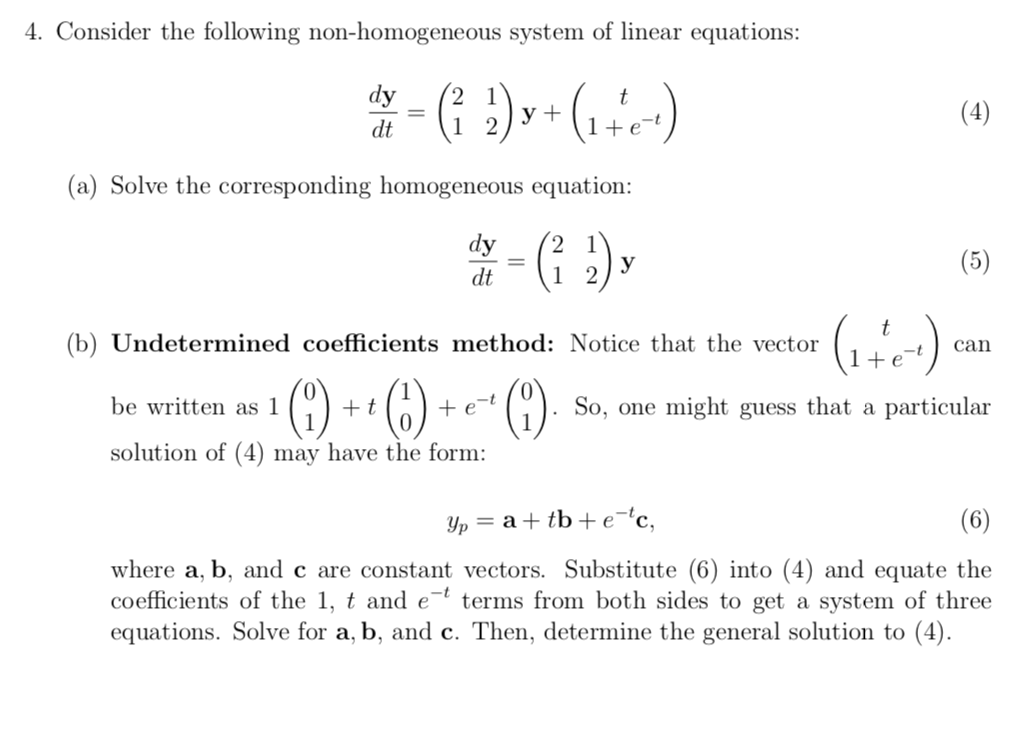
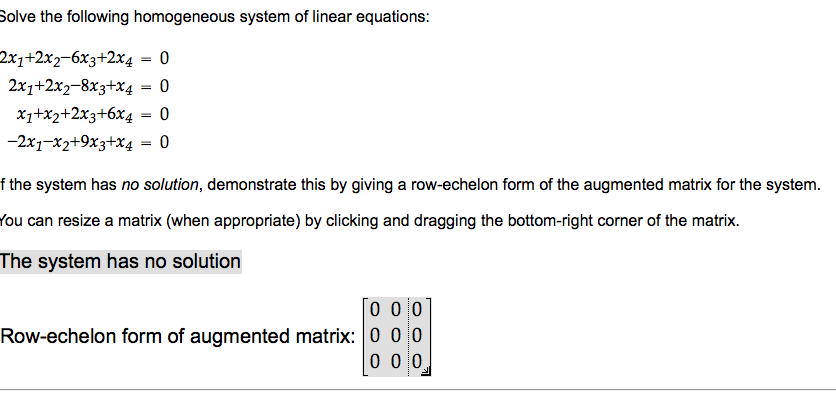
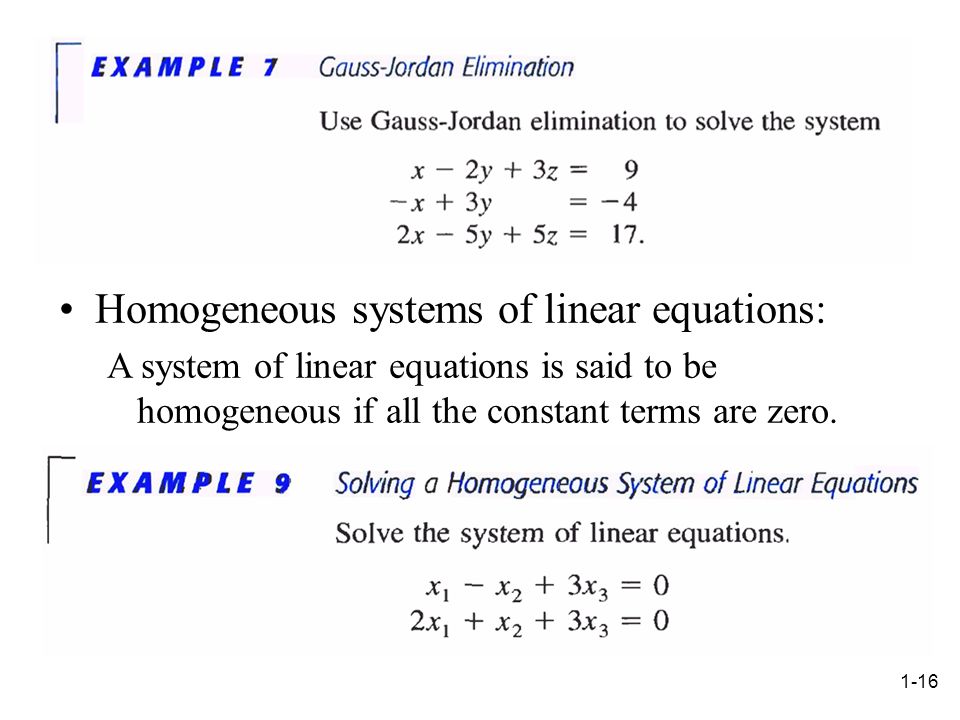
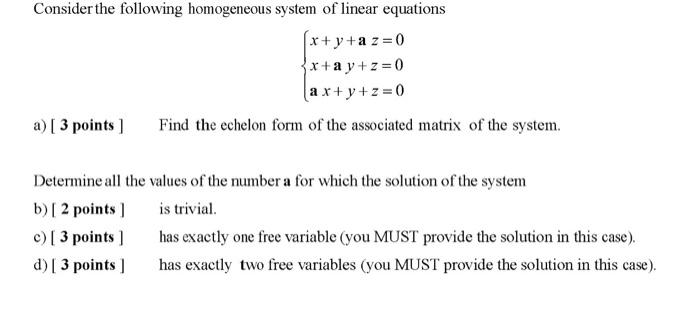
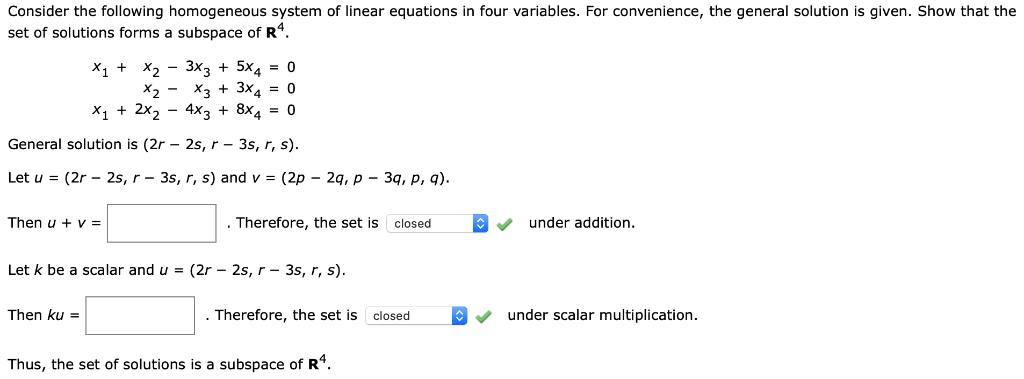
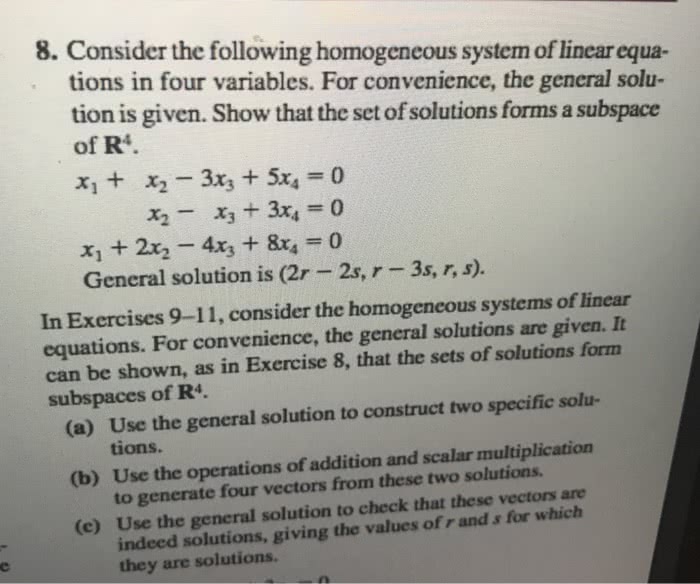
A linear differential equation or a system of linear equations such that the associated homogeneous equations have constant coefficients may be solved by quadrature which means that the solutions may be expressed in terms of integrals. We give an in depth overview of the process used to solve this type of differential equation as well as a derivation of the formula needed for the integrating factor used in the solution process. Equations 2 3 and 4 constitute a homogeneous system of linear equations in four unknowns.

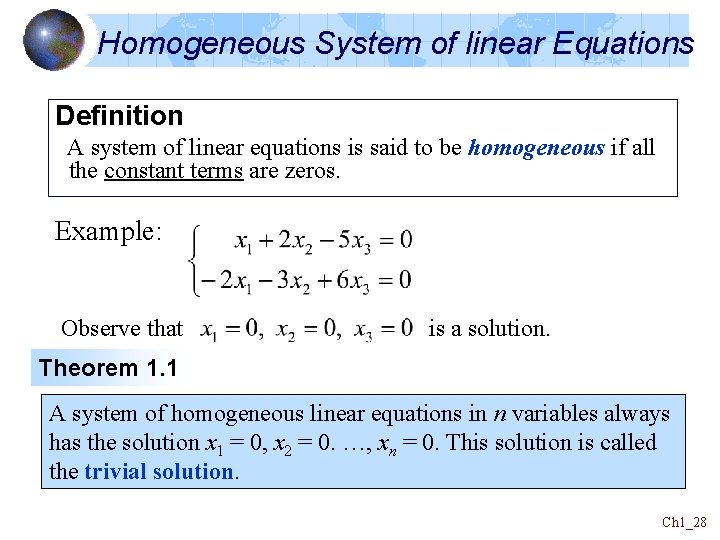
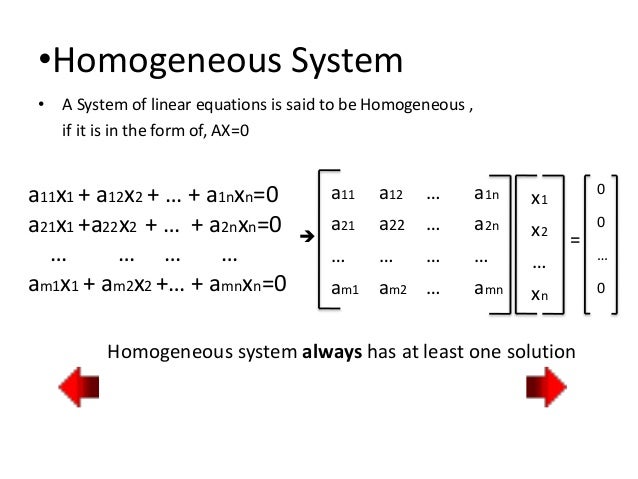
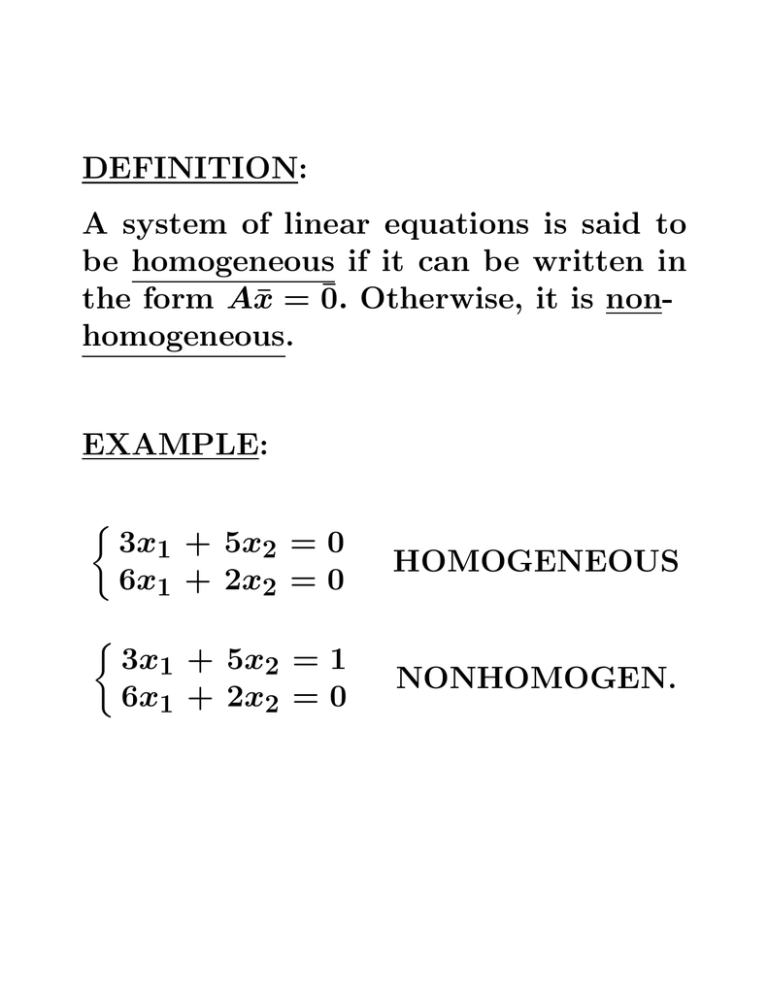
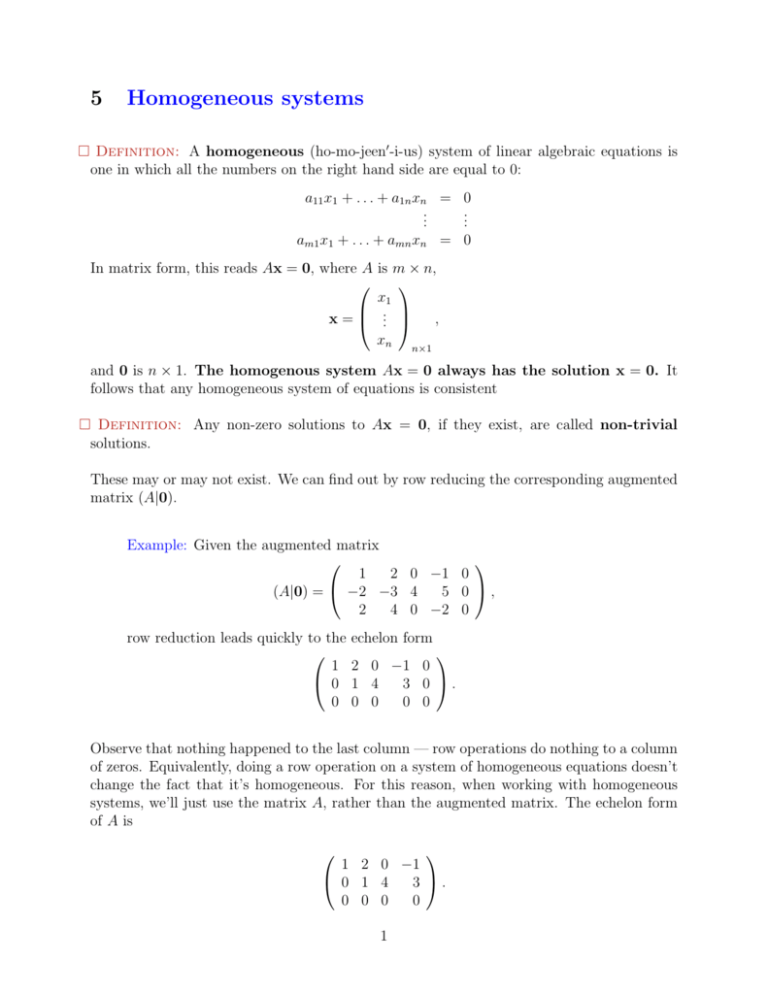
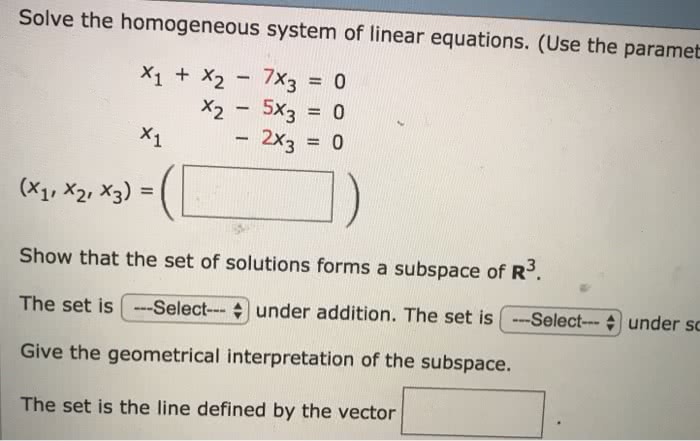
A system of linear equations is said to be homogeneous if the right hand side of each equation is zero ie each equation in the system has the form a 1x 1 a 2x 2 a nx n 0. The solution to a system of linear inequality is the region where the graphs of all linear inequalities in the system overlap. A A is true and R is the correct explanation of A b A is false and R is not a correrct explanation of A c A is true and R is.

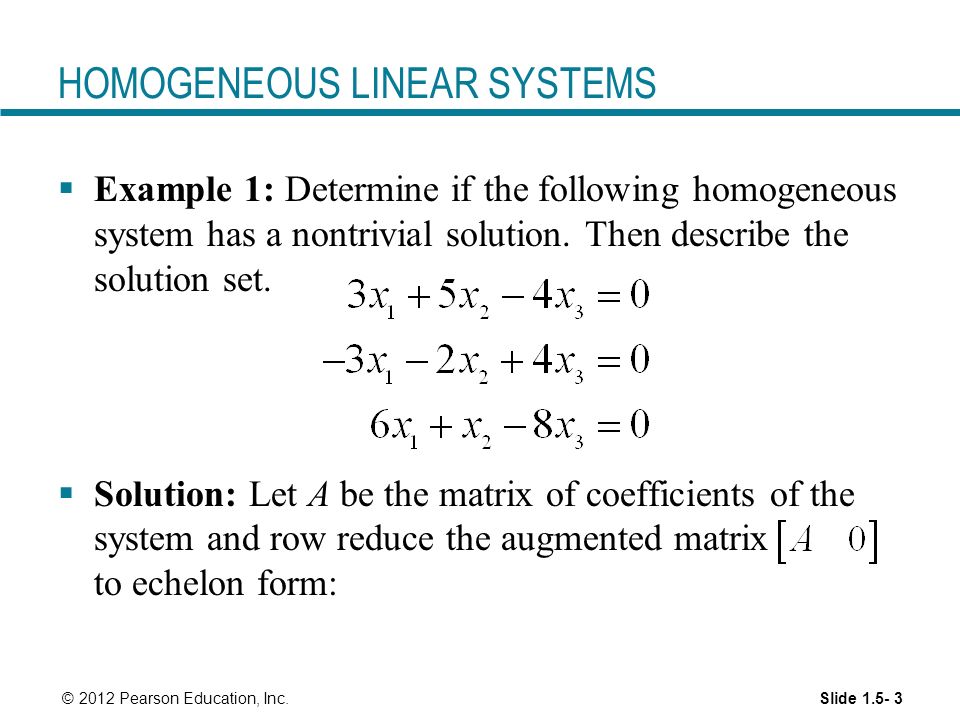
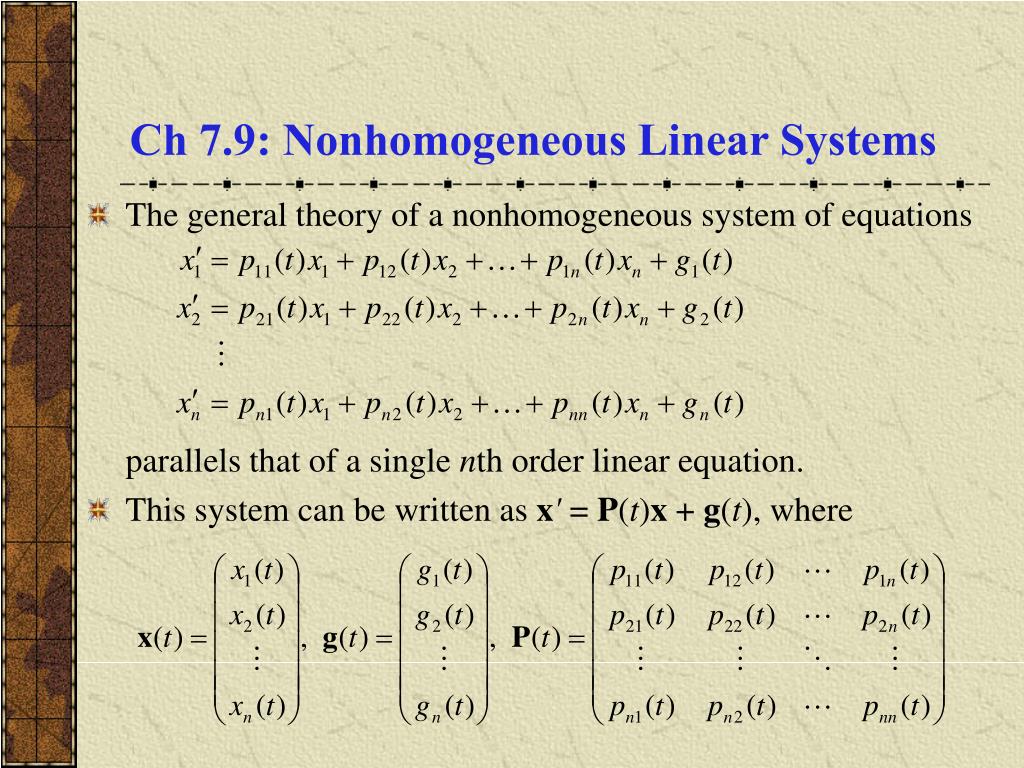
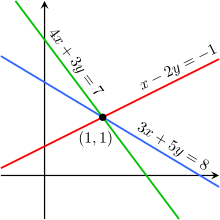
Finding a particular solution to the nonhomogeneous system Ax b. For example is a system of three equations in the three variables x y zA solution to a linear system is an assignment of values to the variables such that all the equations are simultaneously satisfied. Any implicit system which can be divided into system of ODEs which is of the above 4 forms 6.

Therefore for nonhomogeneous equations of the form aybycyrx we already know how to solve the complementary equation and the problem boils down to finding a particular solution for the nonhomogeneous equation. If A. A control system is a system of devices that manages commands directs or regulates the behavior of other devices to achieve a desired result.

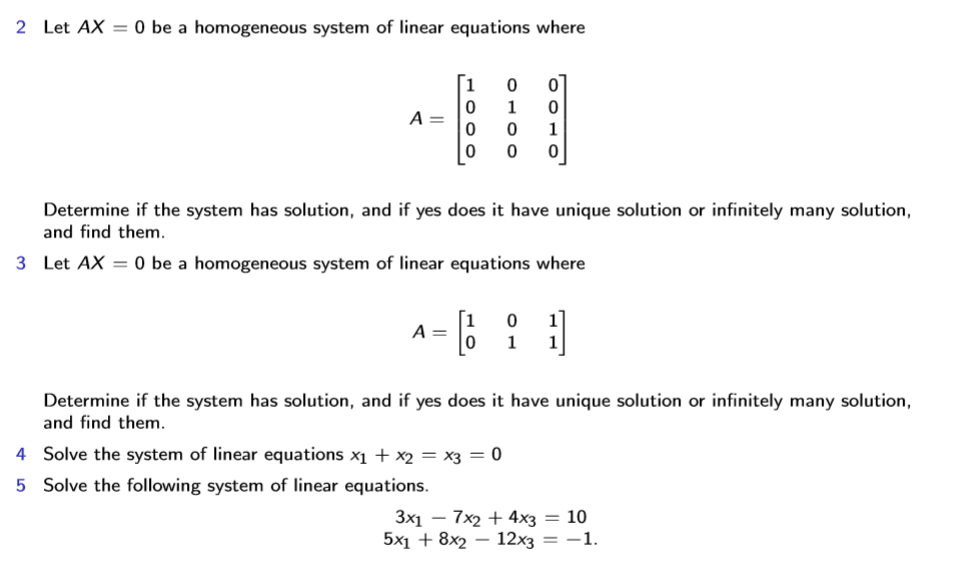
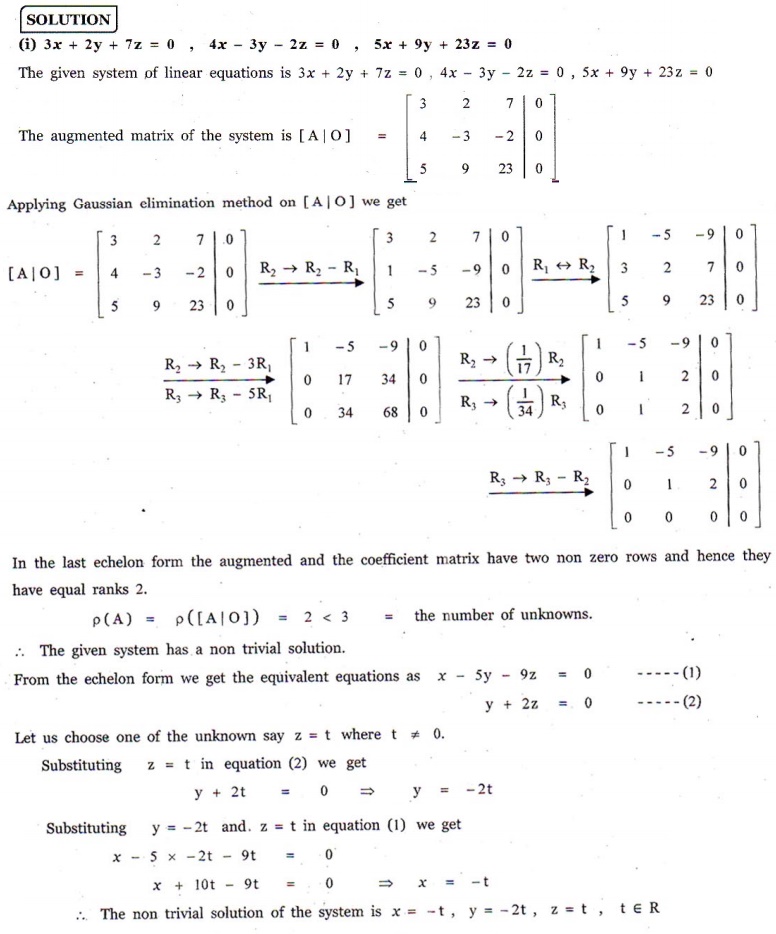
Solving the corresponding homogeneous system Ax 0. In such a case given system has infinite solutions.

Linear Algebra Example Problems Homogeneous System Of Equations Youtube

Homogeneous System Of Linear Equation Youtube

Homogeneous And Nonhomogeneous Systems Of Linear Equations Youtube

Linear Algebra Solution Of Homogeneous System Of Equation Mathematics Stack Exchange

Linear Algebra Proof Of Number Of Solutions In A Linear System What Is Going On Mathematics Stack Exchange

Chapter 1 Systems Of Linear Equations And Matrices

Linear Algebra Lecture 10 Homogeneous Linear Systems Youtube

Ch 7 5 Homogeneous Linear Systems With Constant

Solved 4 Consider The Following Non Homogeneous System Of Chegg Com

Lecture 3 Gauss Jordan Method Homogeneous Linear System

Solved Homogeneous Versus Non Homogeneous Linear Systems A Chegg Com

Linear Algebra Chapter 1 Systems Of Linear Equations

Solved Solve The Following Homogeneous System Of Linear Chegg Com

Linear Equations In Linear Algebra Ppt Video Online Download

Gate Ese System Of Homogeneous Linear Equations Hindi Offered By Unacademy

Solved Roblem Onsider The Infinite System Of Homogeneous Chegg Com

System Of Linear Equations By Diler

19 System Of Non Homogeneous Linear Equations Problem 1 Complete Concept Youtube

Systems Of Linear Equations Tutorial

Linear Algebra Section 1 5 Solving A Homogeneous System Youtube

Systems Of Linear Equations Ppt Download

Iit Jee L28 Solution Of Homogeneous System Of Linear Equations In Hindi Offered By Unacademy

Definition A System Of Linear Equations Is Said To Be

1

Chap 1 Systems Of Linear Equations Ppt Download

Ppt Ch 7 9 Nonhomogeneous Linear Systems Powerpoint Presentation Free Download Id 3212846

Solved Find The General Solution Of The Following Chegg Com

System Of Non Homogeneous Linear Equations

The Homogeneous System Ax 0

Solved 1 8 27 Find All Values Of K For Which The Following Chegg Com

Solved Find Basis For And The Dimension Of The Solution Space Of The Homogeneous System Of Linear Equations X 3x 2x 2 4y 5z A A Basis For The Solution

Boyce Diprima 9th Ed Ch 7 3 Systems Of Linear Equations Linear Independence Eigenvalues Elementary Differential Equations And Boundary Value Problems Ppt Video Online Download

Bank Exams Homogeneous System Of Linear Equation With Examples Offered By Unacademy

System Of Linear Equations Wikipedia

Solved Consider The Following Homogeneous System Of Linear Chegg Com

System Of Linear Equations Wikipedia

Linear Algebra Homogeneous System Mathematics Stack Exchange

Homogeneous Systems Of Linear Equations Trivial And Nontrivial Solutions Part 1 Youtube

System Of Linear Equation Section 1 3 Homogeneous Equations

Solved Consider The Following Homogeneous System Of Linear Chegg Com

Linear Algebra Finding A Basis For And Dimension Of The Homogeneous System Of Linear Equations Youtube

Oneclass Solve The Homogeneous System Of Linear Equations Use The Paramet X1 X2 7x3 0 X2 5x3 0

Solved 2 Let Ax 0 Be A Homogeneous System Of Linear Chegg Com

Oneclass 8 Consider The Following Homogeneous System Of Linear Equa Tions In Four Variables For C

Non Homogeneous Linear Equations Trivial Solution Non Trivial Solution Video Dailymotion

Exercise 1 7 Matrix Homogeneous System Of Linear Equations Problem Questions With Answer Solution

Solved 1 What Is A Homogeneous System Of Linear Equations Chegg Com

System Of Homogeneous Linear Equations

1

We give an in depth overview of the process used to solve this type of differential equation as well as a derivation of the formula needed for the integrating factor used in the solution process.

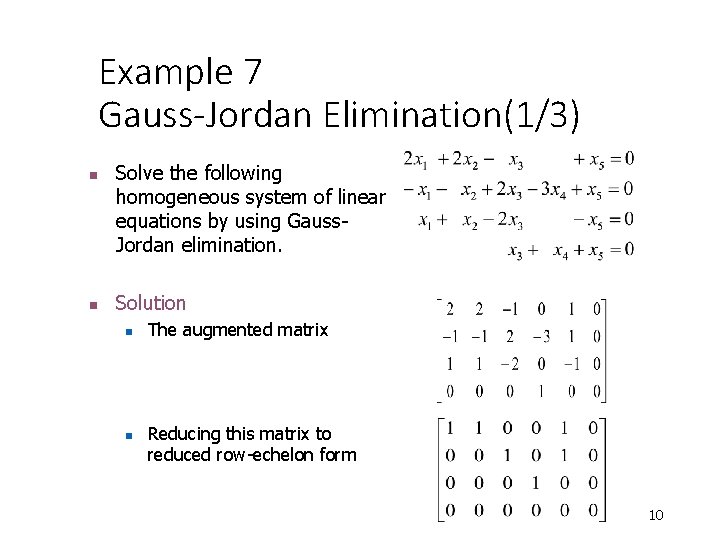
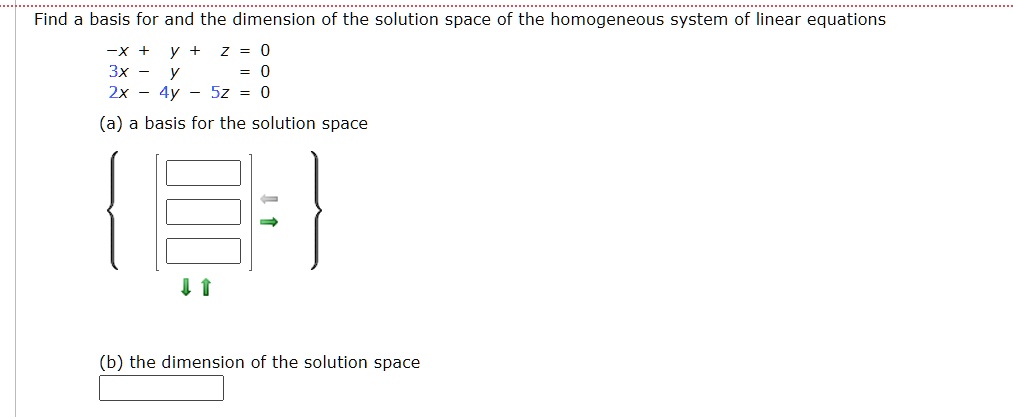
If PAB PA No. C If the system of homogeneous linear equations possesses non-zeronontrivial solutions and Δ 0. Any higher order linear system of ODEs that can be reduced to one of the 5 forms of systems. If PA number of unknowns infinite number of solutions. This returns a basis for the solution space to Ax 0. Do this using the null command by typing nullA. Those systems of two equations and two unknowns only. This is also called trivial solution If PA number of unknowns unique solution. Therefore ρA ρAB 3 4 umber of unknowns.

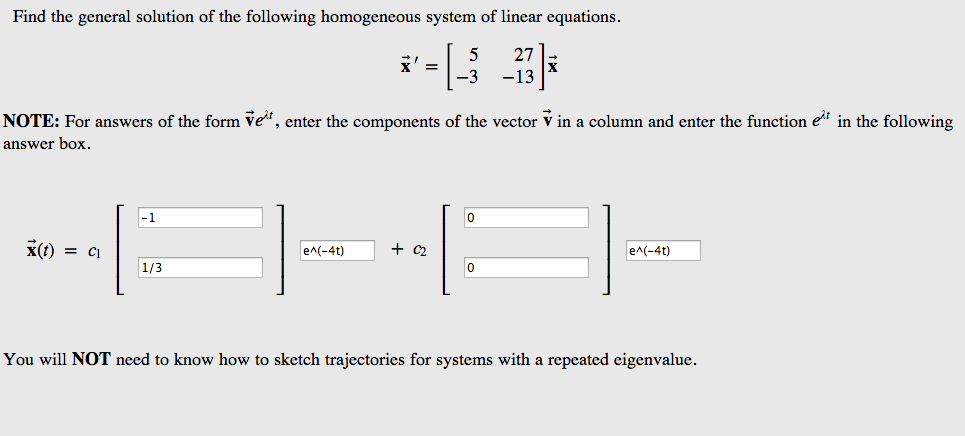
We give an in depth overview of the process used to solve this type of differential equation as well as a derivation of the formula needed for the integrating factor used in the solution process. A homogeneous system of 3 linear equations in 4 unknowns always has a solution in fact always has a non-trivial solution a solution where the unknowns are not all zero. Where a b and c are constants a 0. And the general form of solutions as. Any other solution is a non-trivial solution. If A. The language and ideas we introduced for first order linear constant coefficient DEs carry forward to the second order casein particular the breakdown into the homogeneous and inhomogeneous cases.